12
Personal Experience: A Most Vicious and Limited Circle!?
the case here. An approach often used in analyzing survival data is to apply the
Cox proportional hazard model introduced by Cox (1972). In his approach Cox
devised the hazard function h(t|xj) as a log-linear function of time-dependent
baseline hazard and individual-specific instruments. The hazard function is writ-
ten as
h(t | xj)= h0(t)exp(x jβx)
where t is time, x a vector of covariates and βx a coefficient vector.
The baseline hazard h0(t) varies with time but is independent of any individu-
alistic parameters. Since the baseline hazard is equal for all subjects, there is no
need to specify its shape, so the approach is flexible and easy to compute. The
covariates work as factors of proportionality, that is, they are presumed simply to
shift the baseline hazard curve up or down with the same impact at each point in
time. This functionalism is formulated as the proportional hazard (PH) assump-
tion, which is the key assumption in the Cox model. It follows from this assump-
tion that the hazard of a subject i can be computed by multiplication with the
hazard of subject j. Or, to put it another way, the hazard ratio, which is the quota
of hazard rates between the hazards of subjects i and j, is constant over time
h (t | xi )
hj (t | x1 )
= exp[βxT (xi
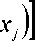
The invariance of covariate effects over time is a very strong presumption. It
is easy to violate this assumption since it reasonable that initial effects of time-
constant covariates actually vanish over time. There are several methods to test
whether the PH-assumption holds. Doing so with regard to the covariates intro-
duced reveals no serious violation of the assumption.4 At least, the Cox likeli-
hood function to be maximized in its simplest form can be derived as
k
L(βx)=∏
j=1
exp( xβ) '
v∑ i ∈ √χp( xβ- ) y
where k is the number of the distinct points in time at which failures occur.
The closure probability analysis is performed sequentially, with each step
considering a more disaggregated closure type. A first regression (model A) in-
cludes all eχit types as eχit events, independent of closure conditions. All types
of eχit are thus considered equivalent in meaning. Model B uses the firm failures
as eχit events. Voluntary closures that do not indicate failure are treated as cen-
sored values. Introduced by Kalbfleisch and Prentice (1980), such an approach is
used in several studies (Harhoff et al. 1998; Kay 1986; Narendranathan and
Stewart 1991; Taylor 1999). In model C bankruptcy is disentangled from the
failure type aggregate and is thus considered as a competing risk to the other
types of closure. The alternative closure events are treated as censored in this
specification. For a summary of the estimated models, see Table 3.
More intriguing information
1. Accurate, fast and stable denoising source separation algorithms2. Herman Melville and the Problem of Evil
3. Errors in recorded security prices and the turn-of-the year effect
4. Evidence on the Determinants of Foreign Direct Investment: The Case of Three European Regions
5. Placenta ingestion by rats enhances y- and n-opioid antinociception, but suppresses A-opioid antinociception
6. The name is absent
7. The Trade Effects of MERCOSUR and The Andean Community on U.S. Cotton Exports to CBI countries
8. Regionale Wachstumseffekte der GRW-Förderung? Eine räumlich-ökonometrische Analyse auf Basis deutscher Arbeitsmarktregionen
9. The name is absent
10. Tourism in Rural Areas and Regional Development Planning