The name is absent

Review of Islamic Economics, Vol. 8, No. 2, 2004

estimated the function with the associated input share equations,
derived using the Shephard’s Lemma, ¹¹ But the difficulty is that their
derivation implies that the PUs are using their long run efficient input
mix, i.e. the firms are assumed allocatively efficient. Hence, most
studies now estimate a single cost function. This is possible because
many PUs, in fact, produce a single output, and others can aggregate
their multiple outputs into a single output index (Kebede, 2001: 13).

In a simple single output and multiple inputs case, we estimate
the frontier using the functional relationships:

^c_lt=f(y,v ^wkt> ⁺ ¾
where ε_it = Vj_t+Uj_t

In (2) C_ll is the total cost of PU_i in a period t, y-_t and ιι>_kt are
vectors of output and input prices respectively f (y_ll ; w∣,_t) provides the
cost frontier. The random disturbance term ε_jt allows the function to
vary stochastically. It has two components: the υ∙_ts are independently
and identically distributed (Hd) elements; they are truly uncorrelated
with the regression. In contrast, the u_jls are non-negative variations
associated with the technical inefficiency of the PU_i. Thus, the error
term e is not symmetric as u∣_t ≥ o.'ⁱ

Figure 2: Results Need not be Identical

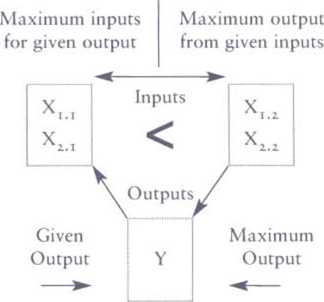
Estimating the PU-Specific inefficiency is the ultimate objective of
the model. This requires the extraction of separate estimates for and

<<   8   9   10   11   12   13   14   >>

More intriguing information