The name is absent

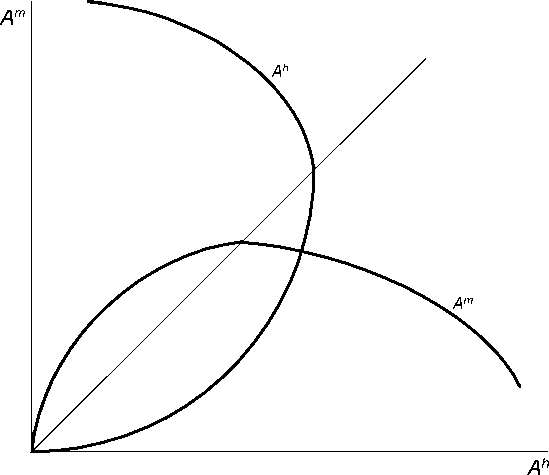
Figure 3. The Shape of Equilibrium Responses

By exactly the same argument, for every A^h there is a unique equilibrium response (A^m, s^m).
We now proceed to describing the overall equilibrium. This analysis depends on the following
useful fact:

OBSERVATION 1. If the equilibrium response A^h is positive, it increases with A^m as long as A^h exceeds
A^m, and declines as A^m increases whenever the opposite inequality holds.⁹

Proving this observation is a simple exercise. By virtue of (3), all we need to do is study
whether C increases or decreases with A^m . For instance, if C increases in A^m, then by the earlier
argument, A^h increases.¹⁰ Using a standard complementarity argument, it is easy to see that
C increases with A^m if the derivative p1 (A^h , A^m) increases with A^m, and decreases otherwise.

Recalling (1), we see that

ψ(Λ^m)ψ'(A^h)
[ψ(A^m) + ψ(A^h)]2^,

so that the derivative increases or decreases with A^m depending on whether A^h is larger or
smaller than A^m .

Observation 1 tightly pins down the shape of the equilibrium response function. Either it
stays flat at 0 throughout — the uninteresting case — or it is “hump-shaped”, initially rising

⁹The zones of increase and decrease will be punctuated by flats corresponding to the jump segments in s; these flats
will be small if the wage rate differential across neighboring types are small. In any case, these have no effect on the
results.

¹⁰It will stay constant with the entire increase transferred to s^h in case we are at a jump segment; see previous
footnote.

<<   6   7   8   9   10   11   12   >>

More intriguing information