A NEW PERSPECTIVE ON UNDERINVESTMENT IN AGRICULTURAL R&D

2. An economic model of the selection of R&D projects

In a strict formal priority setting exercise R&D projects are ranked at the hand of their expected rate
of return (ERR). This ERR is the internal rate of return (IRR) that equalizes the costs of an R&D
project with the benefits resulting from it:
t=n       _∧          t=n       _∧

[1]            ∑C_t ( IRR )^-1 = ∑B_t ( IRR )^-1

t=0                      t=0

where C_t and B_t represent the (expected) R&D costs and benefits in year t, and n the number of years
between the start of the R&D project and the moment that the benefit stream is expected to end.

Not only n differs from project to project, but also the cost and benefit streams themselves
differ in length and shape. Some R&D projects take only one year to complete, while others take 10
years or more (e.g., plant breeding) before a benefit stream emerges. Some technologies are adopted
rapidly and widely, while others are adopted slowly and limited. Assumptions have to be made about
all such aspects to estimate the ERR.

The number of possible R&D projects can be assumed to increase exponentially going from
high to low ERRs, creating an exponential curve (figure 1). Under the assumption of rational
economic behavior and full information, the selection of R&D projects for implementation starts with
the project with the highest ERR and continues with the next highest until the R&D budget has been
exhausted or the ERR on the last (i.e., marginal) R&D project approaches the social cutoff rate,

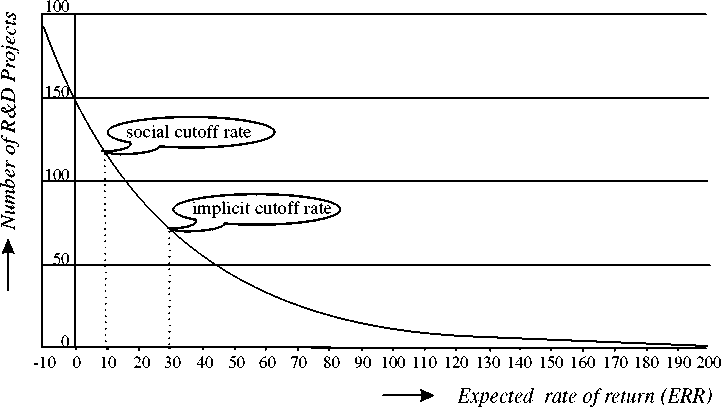
Figure 1: A ranked distribution of R&D projects

<<   1   2   3   4   5   6   7   >>

More intriguing information