The Impact of Optimal Tariffs and Taxes on Agglomeration

loss the final question arises which level of transfer may guarantee a real wage
relation of unity. At the sustain point the necessary level of transfers,RS U S,
can be calulated by setting (20) to one and solving for RSUS to get:

2(1 - TI)(1 - ΘP^γ)

1 - ΘΘ* + TI [ΘΘ*γ - 1 + ΘPy(1 - γ)]

Substituting now the optimal tariffs of (15) yields the necessary amount
of transfers to guarantee a long-run income convergence at L=0. Figure 6
presents this result with the simple outcome that transfers must increase
continuously as integration proceeds and trade costs decrease. Furthermore,
the volume of transfers is considerable higher than the level which has to be
paid to compensate only the welfare loss.

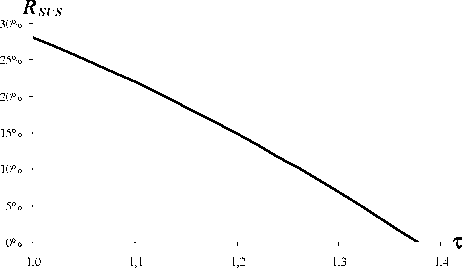
Parameters: γ = 0.5, σ = 4

Figure 6: Necessary transfers (in % of Y ) to guarantee convergence for L=0

8 Conclusion

This paper has shown that within an agglomeration model countries or re-
gions face an incentive for taxing the competitive sector and protecting do-
mestic monopolistic industries by corresponding tariffs. Two cases have been
compared, policies with and without cooperation, while the goal is maximiz-
ing aggregated welfare in the former case and regional welfare in the latter
case. The main result is that under cooperation especially less developed

18

<<   19   20   21   22   23   24   25   >>

More intriguing information