intersection of the reaction curves.5 Assume that in the case of no coordination,
these levels will result.6
The immediate question arises of how to define a unilateral action, since unilat-
eral movement away from a Nash-equilibrium is not a self-enforcing move? In
Hoel (1991), this is accomplished by assuming that one country acts as if it is
not maximizing (1), but instead NBi = Bi (q ) - C (qi ) + h ■ ∑ qi , where h>0 in-
dicates that the country also benefits from reduction in ways not covered by the
cost and benefit relations defined in (1).7 Hoel (1991) stresses that h are not a
choice variable.
Figure 1. The effect of a unilateral increase in reductions by country 1
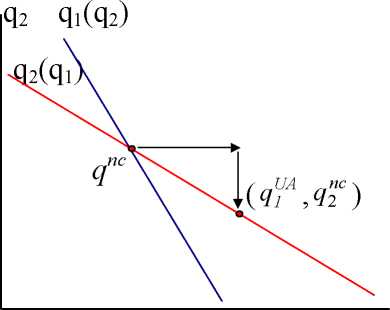
Given the definition given above, what happens in Hoel’s case is easily deter-
∂qi(qj)
mined. Since reductions are strategic substitutes, i.e. ——— < 0, any unilateral
increase in reductions by one country will be responded to by a decrease in re-
ductions by all other countries. This is illustrated in a two-country version, in
5 We occasionally use vector notation Bi(qi,q-nic) , which, due to the assumption of a uniformly
mixed pollutant, is equal to B (q +∑ qnc) .
i i j≠i j
6 This is a common observation; see e.g. Hoel (1997).
7 See Hoel (1991) for a precise definition of h. In this way Hoel defines that any reduction above
the Nash equilibrium is a unilateral action, and at the same time it is in a sense a new stable
situation since there will be no further adjustments.
12
More intriguing information
1. Word Sense Disambiguation by Web Mining for Word Co-occurrence Probabilities2. Sex-gender-sexuality: how sex, gender, and sexuality constellations are constituted in secondary schools
3. Concerns for Equity and the Optimal Co-Payments for Publicly Provided Health Care
4. ‘Goodwill is not enough’
5. Macroeconomic Interdependence in a Two-Country DSGE Model under Diverging Interest-Rate Rules
6. The name is absent
7. The name is absent
8. The name is absent
9. The name is absent
10. An Estimated DSGE Model of the Indian Economy.