W/P
Figure 2 Pecuniary externalities associated with transport system improvements
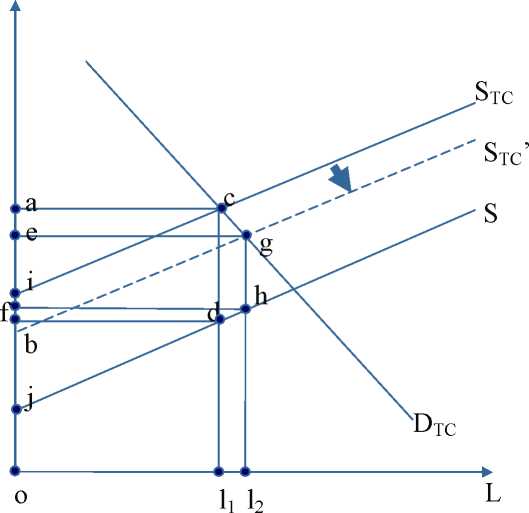
Demand (DTC) for and supply (STC) of labour are the standard curves which cross at
equilibrium, c. Real wages both with and without transport costs, TC are shown in figure 2.
Seen from the viewpoint of the producer and the place of production, the demand for labour
includes transport costs, DTC. There are two supply curves for labour, STC which shows labour
supply in relation to the real wage (W/P) including transport costs (from the point of view of
place of production) and S which shows labour supply in relation to the real wage (W/P from
a place of residence point of view), net of transport costs from place of residence point of
view. It can be assumed, that S is fixed in the long run, whereas STC, which includes transport
costs, will shift with changes in these costs. In the case of transport system improvement the
STC curve shifts to the right (STC’). This leads to a fall in equilibrium wage from a to e, a
change in total wage bill from acl1o to egl2o and a change in expenditure on transport for
commuting from acdb to eghf. Whether demand for transport increases or decreases depends
on the price elasticity of demand for transport. The pecuniary externality for the produces is
acge and for labour fhdb.
Corresponding externalities from the market for transport appear in the market for
commodities, which can be illustrated in a figure similar to figure 3. Equilibrium in the
commodity market is established in market prices, including the cost of transport of
commodities. The demand curve for commodities intersects with the supply curve at market
prices. From the viewpoint of the producer, supply is determined in basic prices, net of
transport costs. Supply in market prices is determined by the use of an adding-on principle. A
transport system improvement will reduce transport costs and will shift the supply curve in
market prices to the right. Equilibrium prices fall, which affects the real wage (w/p). Thus, a
change in transport costs will affect both w and p as shown on the vertical axis of figure 3.
However, it is reasonable to assume that for any given decrease in transport costs, w will fall
More intriguing information
1. Neighborhood Effects, Public Housing and Unemployment in France2. The name is absent
3. A methodological approach in order to support decision-makers when defining Mobility and Transportation Politics
4. Qualification-Mismatch and Long-Term Unemployment in a Growth-Matching Model
5. The name is absent
6. Keynesian Dynamics and the Wage-Price Spiral:Estimating a Baseline Disequilibrium Approach
7. Inflation Targeting and Nonlinear Policy Rules: The Case of Asymmetric Preferences (new title: The Fed's monetary policy rule and U.S. inflation: The case of asymmetric preferences)
8. The name is absent
9. The geography of collaborative knowledge production: entropy techniques and results for the European Union
10. Graphical Data Representation in Bankruptcy Analysis