The name is absent

Table 1. The data set: summary statistics (reference year: 1998)

Notes:

^a The firms in the sample are in manufacturing and larger than 50 employees

^b Source OECD STAN database, OECD , 2001

^c OECD (2001), Measuring Globalisation, Volume I: Manufacturing Sector, OECD Paris, 2001

The coverage of the final data set of national manufacturing activities varies by country, from
40.33% in Sweden to 5.68% in Italy. Also, MNEs are over-represented compared to their share in
national manufacturing employment, mainly because of the exclusion of firms smaller than 50
employees. However, average output per employee and average cost of employment per employee for
NEs and MNEs in the sample (Table A1) are in line with those at the population level, according to
OECD data. Consistently with most of the available evidence, MNEs in the sample report higher
output per employee and employment costs per employee than NEs. This gap, which is robust when
we control for size and sector distribution (unreported), is possibly related to higher skill intensity in
MNEs. Regrettably, in our sample we cannot directly measure this effect, as we have no information on
the skill composition of employment.

3.2. The econometric model and results

We are interested in measuring both the speed and the extent of labour demand adjustment across firms.
We derive from a Cobb Douglas production function a constant-output dynamic labour demand
function for a generic cost-minimising firm under the assumption of partial adjustment of the type
λ

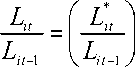
, where 0 ≤ Λ≤ 1, while L_it and L_it are, respectively, effective and desired employment

in firm i at time t. In logarithmic form, the firm-level conditional labour demand is derived as follows,

8

<<   6   7   8   9   10   11   12   >>

More intriguing information