346
N K Humphrey, G R Keeble
3 Results and interpretation
The results of experiment 1 (showing the effect of colour) are given in figure За, and
of experiment 2 (showing the effect of noise) in figure 3b. The data are presented
in the form of log ‘survivorship curves’ in which the proportion of bouts which lasted
at least t s is plotted on a log scale on the ordinate against t on the abscissa. Each
figure shows the mean for all seven monkeys; because the ordinate has a log scale
the mean is represented by the geometric rather than the arithmetic mean of the
individual monkeys’ data.
The shape of these curves is remarkable: they clearly approximate straight lines.
Admittedly these are averaged data, but inspection of the results from the individual
monkeys shows that they all followed the same linear pattern.
Let us consider first what these straight lines signify, before discussing the
influence of colour and noise. A straight line on a log survivorship curve implies
that the individual bout lengths conform to a Poisson distribution: the probability
that a bout, which has already lasted for time t, will be terminated between time t
and t+δt is constant and independent of t. For example the probability that the
monkey, having stayed for 25 s, will leave before 30 s is exactly .the same as the
probability that the monkey, having stayed for only 5 s, will leave before 10 s. Such
a distribution is characterised by its ‘half-life’, which is equal to the time within which
half the surviving bouts may be expected to be terminated. The half-life is inversely
proportional to the slope of the straight line.
The meaning of this kind of distribution may be explained by a simple model.
Suppose that every h s the monkey were to toss a coin: if the coin comes down
heads the monkey moves, if it comes down tails the monkey stays where it is—and
h s later tosses the coin again. Then sometimes the monkey will move after the first
toss, but sometimes it will wait for several tosses before doing so. If each coin toss—
and thus each decision to move or stay—is genuinely independent of all earlier ones,
the bout lengths will be distributed according to a Poisson distribution. On the
assumption that at each toss there is a 50% chance of the coin coming down heads or
tails, then h will be the half-life of the distribution.
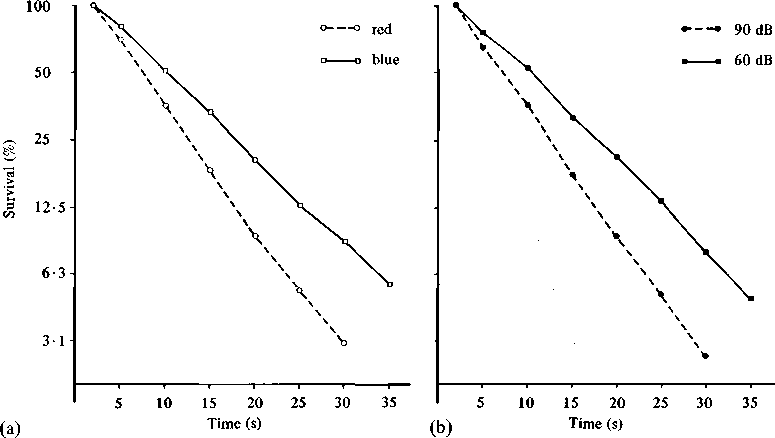
Figure 3. Log survivorship curves for bouts in (a) red light and blue light (68 dB noise);
(b) 90 dB and 60 dB noise (white light).