POWER LAW SIGNATURE IN INDONESIAN LEGISLATIVE ELECTION 1999-2004

one. The difference of statistical facts between election 1999 and 2004 is only about
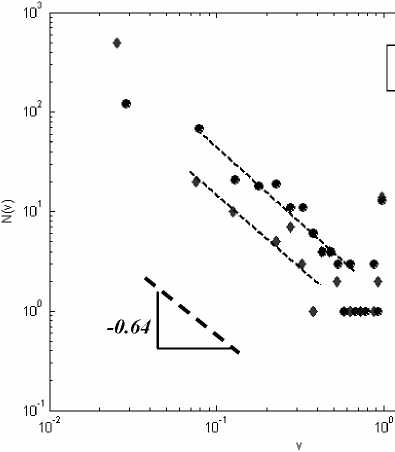
other coefficient of the distribution equation. As described in fgure 2, it is clear that the
the fitting power distribution of 2004 election is laid above the 1999 one.

♦ DPR 1999

• DPR 2004

Figure 2

The power-law signature in the election result for DPR (House of Representative ) in 1999 and
2004.

3. Discussions

In general, the power law distribution can be written as

N(v) ≈ Cv^-α

or in double-logarithmic form

log N (v) ≈ logC -αlogv          (7)
hence we have a straight line fit in the double-log scales. Statistically, this means that

P_r[V =v]~v^-α

the probability of a candidate gains v votes equals to v^-α, and in this specific case, the
exponent goes around the unity (α ≈ 1), as described in the previous work (Situngkir &
Surya, 2003).

An important lesson we can get from this fact is that social dynamics representing by the
the statistical properties of election result are a self-organized criticallity. Every system
(including social system) will be persistently evolve towards the critical state. The critical
state is signatured by the response functions depict no characteristic scales (Bak, 1997).
The power-law distribution is scale-free, means that the character of the critical system
does not depend upon time and distance. A sign that showed the robust character of
critical state, specifically in social system.

<<   1   2   3   4   5   6   >>

More intriguing information