50
. Time to compute reduced model using IRKA
A1000r
Output error (IRKA vs. quasi-active)
---h = 2 μm (N = 41364)
---h = 0.5 μm (N = 165330)
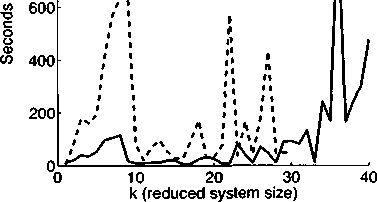
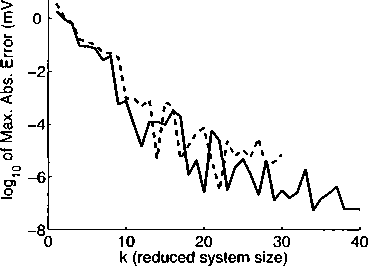
Figure 2.13: A) Time to compute the reduced system, and B) maximum absolute error between
the quasi-active and reduced system output using IRKA on cell n408. Solid lines correspond to
the N = 41364 case, while dashed lines are for the N = 165330 case. In (A) the sudden drop in
computation time around к = 10 occurs for both cases because at that point, though the shifts have
not converged, the reduced system is accurate to a tolerance of 3 digits or more
the soma is the site of action potential generation, we only need to check whether
y(t) = υσ{t) ≥ Vth
(2.44)
where V⅛ ɪs some threshold voltage (recall that vσ(t) ≡ v1(∕bι,t)). At each time-
step during the simulation we check if threshold was reached, and if so then we
instantaneously reset the soma voltage to the rest and hold it there for a refractory
period of τref ms.
2.7.1 Thresholding at the Soma
Reset values should be chosen to produce as much similarity as possible between
the outputs of the active and quasi-active models while still being biophysically rea-
More intriguing information
1. GROWTH, UNEMPLOYMENT AND THE WAGE SETTING PROCESS.2. The name is absent
3. Learning-by-Exporting? Firm-Level Evidence for UK Manufacturing and Services Sectors
4. The name is absent
5. Computational Batik Motif Generation Innovation of Traditi onal Heritage by Fracta l Computation
6. Migration and Technological Change in Rural Households: Complements or Substitutes?
7. Qualifying Recital: Lisa Carol Hardaway, flute
8. The name is absent
9. TWENTY-FIVE YEARS OF RESEARCH ON WOMEN FARMERS IN AFRICA: LESSONS AND IMPLICATIONS FOR AGRICULTURAL RESEARCH INSTITUTIONS; WITH AN ANNOTATED BIBLIOGRAPHY
10. An alternative way to model merit good arguments