Imperfect competition and congestion in the City

dPi^w ∂²∏i(w_i,w-i,p)
dwi      ∂w_i²

1-

FOC

( 2 (⅛)'

+ Pi^w

d²Pi^w
dw²

dgi(wi)A + dPj^w d d²gi(^wi)P_w
dwi J    dwi I dw2 ⁱ

- 2Λ^h

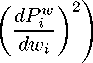
We wish to show that this expression Ω is negative given that dPW / dwi > 0.
To to that, when there is no ambiguity, in order to simplify expressions, we use
the following notations:

P≡ Pi^w = Pi^d

P о ≡ dΡW
dwi

_P00 ≡ d²PW
dw2
i
0 _ dgi(wi)
g      dwi

00 _ d²gi(wi)
g ≡  dw²

Using these notations, we have equivalently:

Ω = (-2 (P⁰)2 + PP"ɔ (1 - g⁰) + P⁰ (g⁰⁰P - 2Λ^h (P⁰)²} .       (59)

We now need to compute P⁰, P⁰⁰, g⁰ and g⁰⁰ at any point (i.e. not only at the
symmetric candidate equilibrium).

First let compute P⁰ and P⁰⁰. Recall that

P = P_i^w

exp

∕wi -Λ^w pw

∖   μ^w

/Wj∙ -Λ^wPj^wλ
ex^{p eχ}p( —μw^j)
1...n

We have, using again the implicit function theorem

μW ^p (1 — ^p )

1 + λw P (1 - P ).

Note that:

∂P

Pо      ∂wi

P = i _ ∂P

¹   ∂P^w

[P (1 - P)]⁰ = (1 - 2P) P⁰

Therefore, after simplifications, we get:

32