The name is absent

Stata Technical Bulletin

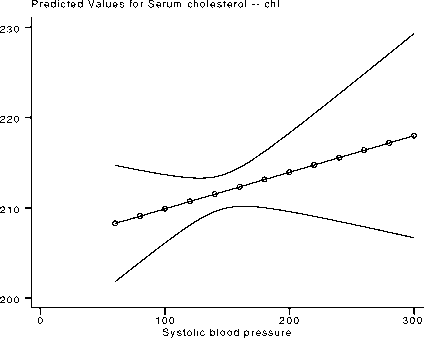
Figure 1

In this specification, neither age nor systolic blood pressure affects the cholesterol level. But suppose that the systolic blood
pressure variable is jointly determined with the cholesterol level, chi, such that each affects the other. In that case, the ordinary
least squares estimator of the impact of sbp is biased and one must use two-stage least squares, also known as instrumental
variable estimation. Suppose that the socioeconomic status of the subject (ses in the evans2.dta dataset) is thought to be
correlated with sbp but not a function of chi and not a direct influence on chi. In that case, ses can be used as an instrument
for sbp.

This revised specification of the model can be estimated as follows:

. regpred2 chi sbp, f(60) t(300) i(20) adj(age smk) inst(age smk ses)
> level(90) xlabel ylabel

Predicted Values and 90% Confidence Intervals

Outcome Variable:     Serum cholesterol — chi

Independent Variable: Systolic blood pressure — sbp

Covariates:           age smk

Instruments:          age smk ses

Variables set to Zero:

Variables set to One:

Total Observations:   1218

Confidence interval: 90

<<   2   3   4   5   6   7   8   >>

More intriguing information