Figure 7: Lake Dynamics for b=0.48
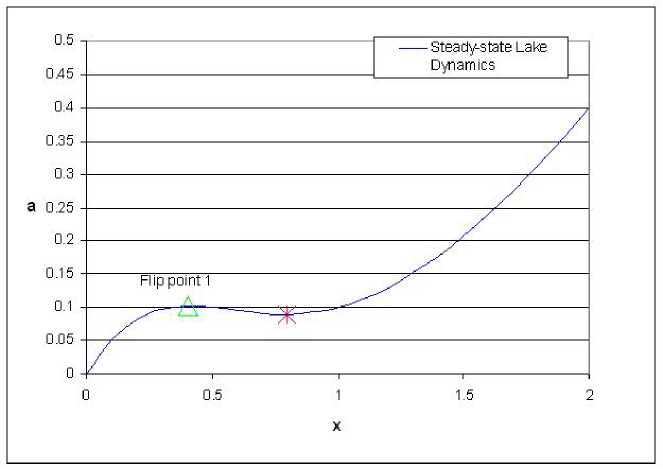
Figure 8: Lake Dynamics for b=0.6
• 2 ≤ b ≤ 8 √3
For values of b in this range, a has three equilibria. (See Figure 8)
This is the hysteresis effect: the lake remains in an oligotrophic state
up to a certain point at which it flips to a eutrophic state. The point at
which the lake flips from oligotrophic to eutrophic is where da/dx = 0 at
(xF1, aF1) = (0.4084, 0.1021) and is an unstable steady-state. The point
(x, a) = (0.7876, 0.0897) is a stable eutrophic state. This hysteresis allows
eutrophication to be reversible, however, as lowering external loading be-
26
More intriguing information
1. The Importance of Global Shocks for National Policymakers: Rising Challenges for Central Banks2. Quality Enhancement for E-Learning Courses: The Role of Student Feedback
3. Getting the practical teaching element right: A guide for literacy, numeracy and ESOL teacher educators
4. IMMIGRATION AND AGRICULTURAL LABOR POLICIES
5. AN EXPLORATION OF THE NEED FOR AND COST OF SELECTED TRADE FACILITATION MEASURES IN ASIA AND THE PACIFIC IN THE CONTEXT OF THE WTO NEGOTIATIONS
6. The name is absent
7. THE CHANGING RELATIONSHIP BETWEEN FEDERAL, STATE AND LOCAL GOVERNMENTS
8. Distribution of aggregate income in Portugal from 1995 to 2000 within a SAM (Social Accounting Matrix) framework. Modeling the household sector
9. Ventas callejeras y espacio público: efectos sobre el comercio de Bogotá
10. Short Term Memory May Be the Depletion of the Readily Releasable Pool of Presynaptic Neurotransmitter Vesicles