Mean Variance Optimization of Non-Linear Systems and Worst-case Analysis

Appendix

Proof of Proposition 1

Using Leibniz’s rule and (1), from (9) we obtain the first order conditions with respect to
the choice variables r^L and Ri :

i

∂Π

--i = r^D(Li + Ri)² - rIl2 = 0, for i = 1...N.                   (14)

Solving (14) for Ri gives

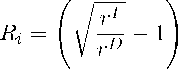
(15)

Solving (13) for r_i^L in a symmetric equilibrium where r_i^L = r_s^L_q for i = 1...N after substituting
(2) and (15) gives

^{l +} (^rLq ^{- c -} √^rI^rD^)(—YN-^--1 ) = ^0,

from which r_s^L_q and c_sq follow. Substituting then r_s^L_q in (2) gives L_sq, and through (15) R_sq.

Substituting R_sq and L_sq in (1), we obtain D_sq.                                    Q.E.D.

Proof of Corollary 1

Solving (3) and (4) gives φi = 1 — DRi and ωi = (R) Ri + Di. Substituting the expressions
for R_sq and D_sq, we obtain φ_sq and ω_sq as in the corollary.                         Q.E.D.

Proof of Lemma 1

We proceed in two steps. First, we show that the variance of the liquidity demand x_m of
the merged banks is minimized when deposits are raised symmetrically in the two regions.
Second, we show that the expected liquidity needs of the merged banks (and therefore their
refinancing costs) are lower when deposits are symmetric.

Step 1. Define the liquidity demand of the merged banks as

x_m = δ1αD_m + δ2(1 - α)D_m,

where α ∈ [0, 1] indicates the fraction of deposits that the merged banks raise in one region
and (1 - α) the fraction they raise in the other region. Since δ1 and δ2 are independent and
V ar(δ1) = V ar(δ2), the variance of xm is simply

Var(x_m)=α²D_m² Var(δ1)+(1- α)²D_m² V ar(δ2)

= V ar(δ1)[α²D_m² +(1- α)²D_m² ].

29

<<   28   29   30   31   32   33   34   >>

More intriguing information