Neural Network Modelling of Constrained Spatial Interaction Flows

M -1(xu, yu ) with u = 1,..., U}, so that g (xu ) = y^u. The set M is the set of input and
output vectors. The task is to find a continuous function that approximates M. In real
world application, U is generally a small number and the samples contain noise.

The Generic Neural Spatial Interaction Model

In the unconstrained case the challenge is to approximate the real-valued interaction
function g(r, s_j, f_jj j : ¾³ →¾, where the 3-dimensional euclidian real space is the
input space and the 1-dimensional euclidian real space the output space. In practice
only bounded subsets of the spaces are considered. To approximate g, we consider the
class Ω of feedforward neural network models with three input units, one hidden layer
that contains H hidden units and a single output unit. The three input units represent
measures of origin propulsiveness, destination attractiveness and spatial separation. The
output unit, denoted by y, represents the estimated flow from i to j. Formally the
neural network model for the unconstrained case of spatial interaction may be written in
its general form as:

{ Ω ; ¾³

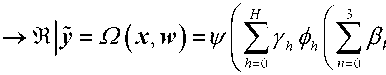
^χ_n   ^{, x} ≡

Vector x = (x0, x₁, x2, x₃ ) is the input vector augmented with a bias signal x₀ that can
be thought of as being generated by a dummy unit whose output is clamped at 1.
Models belonging to Ω(x,w) may have any number of hidden units (H = 1,2,...) with
connection strengths from hidden to the output unit represented by Гh . ^{The β}hn
represent input-to-hidden connection weights. The symbol
w = (wk: | k = 1,...,K = (5H ⁺1)) is a convenient short hand notation of the (5H +1) -
dimensional vector of all the β_hn and γ_h network weights and biases. φ_h and ψ are
arbitrarily differentiable, generally non-linear transfer functions of the hidden units and
the output unit, respectively.

<<   5   6   7   8   9   10   11   >>

More intriguing information