27
Number of Measurements (M)
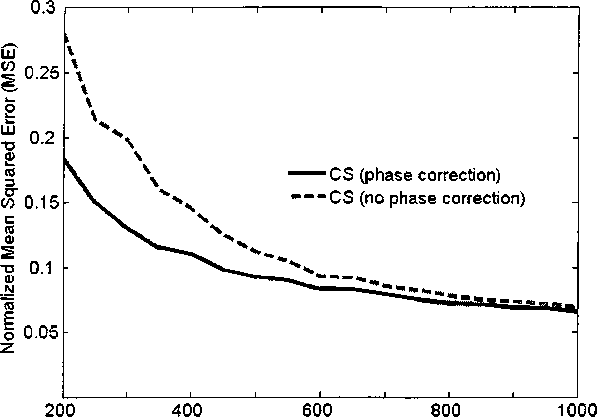
Figure 3.3 : Comparison of quality of image reconstruction between CS with and with-
out phase correction. As the number of measurements (M) used in CS increases, the
mean-squared error (MSE) between the magnitudes of the reconstructed image and
the reference image (see Fig 3.2(a)), normalized by the energy of the reference image,
decreases. CS with phase correction shows superior reconstruction performance.
an object with a constant phase profile has a more sparse representation in wavelet
basis than one with a spatially varying phase profile, CS reconstruction using phase
correction yields superior performance for a given number of measurements.
3.2.3 CS and phase retrieval
If the object mask in Figure 3.1 moves away from the object plane, the acquired
Fourier data will have the correct Fourier magnitude but a distorted phase. No longer
can one use the phase data for image reconstruction. However, an image can still
be reconstructed by combining CS with the well-known technique of phase retrieval
(PR) [67]. Moravec et al. recently developed a PR minimization algorithm, called
More intriguing information
1. An institutional analysis of sasi laut in Maluku, Indonesia2. Before and After the Hartz Reforms: The Performance of Active Labour Market Policy in Germany
3. Une nouvelle vision de l'économie (The knowledge society: a new approach of the economy)
4. The name is absent
5. L'organisation en réseau comme forme « indéterminée »
6. Social Irresponsibility in Management
7. Julkinen T&K-rahoitus ja sen vaikutus yrityksiin - Analyysi metalli- ja elektroniikkateollisuudesta
8. Regulation of the Electricity Industry in Bolivia: Its Impact on Access to the Poor, Prices and Quality
9. The name is absent
10. Wirkung einer Feiertagsbereinigung des Länderfinanzausgleichs: eine empirische Analyse des deutschen Finanzausgleichs