The name is absent

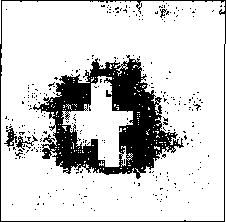
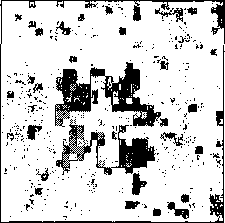
(a) μ = 1                   (b) μ = 5                  (c) μ = 10

Figure A.l : Test FTVd reconstruction results of the Chinese character “light” for
the single-pixel CS imaging system for various μ and a maximum β of 1024. A smaller
μ yields a smoother image while a larger μ gives a sharper but noisier image.

rr-subproblem with the fast Fourier transform, applicable only when Φ is a Toeplitz
matrix. Since the measurement matrix Φ used in the single-pixel imaging system is
random, my implementation uses the matrix left division in Matlab to solve the x-
Subproblem. This implementation can be slow, but works for the small-size problem
in this thesis. The TVAL₃ algorithm should be an faster alternative for solving the
reconstruction problem in (A.l) for general matrix Φ and even for complex data [73].

A quick note on choosing the optimization parameters, μ and β: The regulariza-
tion parameter μ controls the weights between the smoothness (min-TV) assumption
and the measured data used for the reconstruction. A smaller μ yields a smoother
image while a larger μ usually gives a sharper but noisier image because the mea-
sured data is noisy (see Figure A.l). The FTVd algorithm uses a continuation for
β (gradually increasing with the number of iterations), so it only requires an upper
limit for β. For the test results used in this thesis, typical values for μ is between 1
and 10, whereas beta should be powers of 2, between 256 and 1024.

<<   79   80   81   82   83   84   85   >>

More intriguing information