Chapter 4
[SiO]⅝(H*)exp(-⅛
[SiOH] =-------------⅛-
[4.23]
ʌ^sɪ-
[АЮ’] =
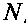
[4.24]
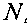
[¾(H*)exp(-⅛J2
[A1OH^] =
a, (H÷ )exp(- ≤)+—J— [¾ (H * )exp(⅛]2
^A1+-^A1-
[4.25]
[SiO- ] =----------------ʒ-ɪ;--------------r- [4.26]
1 + W-¾ + τr∏^[^ (H+)exp(-⅛]2
ʌsi- ʌ'ɪ ʌsi+^-
[SiOHj] =
Wsi
½
[⅝(H*)exp(-⅛]2
[¾(H∙)exp(-⅛2
[4.27]
Here K(Ka∣i, Ka∣2. Ksm and Ksi2) /V (Wai. Afa and Λ∕b) are equilibrium constants
and surface sites densities, respectively, which can be obtained by fitting with the
experiment data. From Eqs. [4.19] - [4.27], zeta potential ζca∣ can be calculated
from the initial evaluates of K and /V. Parameters estimation was done using
Matlab optimization toolbox. The object function to be determined is ∣∣ <ca∣ - ζe×p Il-
Iteration terminates when ∣∣ ζca∣ - ζe×p ∣∣< tolerance.
Figure 4.18 shows the experiment data and fitted curve of kaolinite zeta
potentials in 0.05 M NaCI brine at different pH. Zeta potential of kaolinite becomes
124
More intriguing information
1. The name is absent2. The name is absent
3. ENVIRONMENTAL POLICY: THE LEGISLATIVE AND REGULATORY AGENDA
4. ARE VOLATILITY EXPECTATIONS CHARACTERIZED BY REGIME SHIFTS? EVIDENCE FROM IMPLIED VOLATILITY INDICES
5. Second Order Filter Distribution Approximations for Financial Time Series with Extreme Outlier
6. Legal Minimum Wages and the Wages of Formal and Informal Sector Workers in Costa Rica
7. Novelty and Reinforcement Learning in the Value System of Developmental Robots
8. The name is absent
9. Behaviour-based Knowledge Systems: An Epigenetic Path from Behaviour to Knowledge
10. The InnoRegio-program: a new way to promote regional innovation networks - empirical results of the complementary research -