The technological mediation of mathematics and its learning

Published in Nunes,T (ed) Special Issue, ‘Giving Meaning to Mathematical Signs: Psychological,
Pedagogical and Cultural Processes‘ Human Development, Vol 52, No 2, April, pp. 129-

this situation many times in our iterative design process: solving one problem of
representation threw up a new problem.

Our solution was to invent the idea of shrinking digits. Digits are displayed in
gradually decreasing size until they reach the size of a pixel. In this way the idea that
an infinite number of digits follow the decimal point is conveyed visually. By using
the ToonTalk ‘pumping‘ tool for increasing the size of an object, a student can view
more and more of the digits that initially were too small to see. This process can take
place indefinitely: there is a theoretical size limit based on the memory of the
computer, although there is nothing to stop the process being transferred to a second
computer when the memory is full! Figure 4 provides an illustration of a decimal
representation of the rational number ⁵/49.

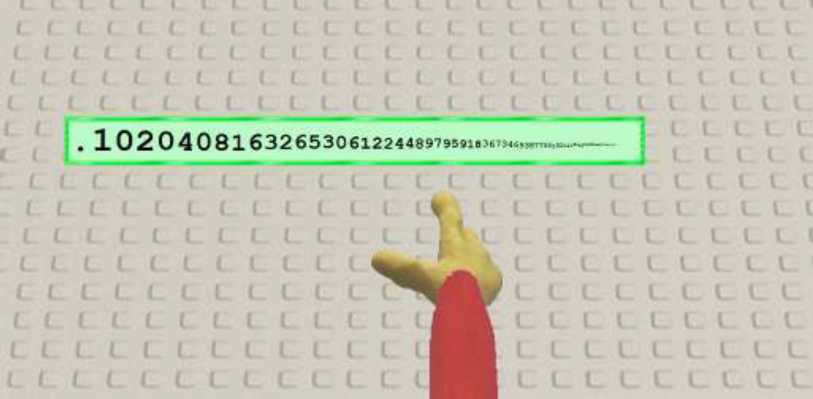
Figure 2. An example of the new shrinking digit display, showing the result of dividing 5 by
49. (You can move ‘your hand‘ to the right, hover over the tiny digits and then pump them up to a size
large enough to read)

Dividing the infinite shrinking digit representing ⁶/7 by ²/7 really does return the exact
value 3.

Our evidence as to the extent the new representational infrastructure enhanced
the mathematical meanings developed by students when compared with the meanings
developed, in paper and pencil, is mixed. We were unable to undertake any large scale
trials due to constraints of technology access and time - inevitable in such
experimental situations - but we did have existence theorems: instances of students
engaging with and undertaking tasks that would, we think, have been impossible

15

<<   12   13   14   15   16   17   18   >>

More intriguing information