Stable Distributions

1.3 Definitions and basic characteristics

Dependence on beta

Gaussian, Cauchy, and Levy distributions

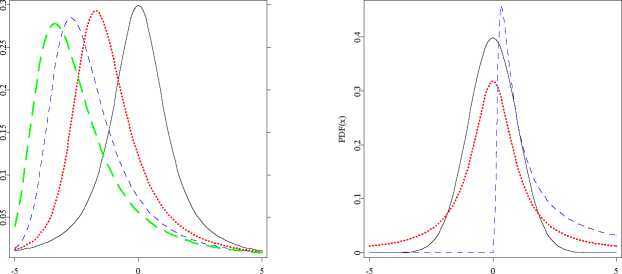
Figure 1.2: Left panel: Stable pdfs for α = 1.2 and β = 0 (black solid line), 0.5
(red dotted line), 0.8 (blue dashed line) and 1 (green long-dashed
line). Right panel : Closed form formulas for densities are known
only for three distributions - Gaussian (α = 2; black solid line),
Cauchy (α = 1; red dotted line) and Levy (α = 0.5, β = 1; blue
dashed line). The latter is a totally skewed distribution, i.e. its
support is R+. In general, for α < 1 and β = 1 (-1) the distribution
is totally skewed to the right (left).

θ STFstab02.xpl

The S^,°, (σ,β,μ0) parameterization is a variant of Zolotariev’s (M)-parameteri-
zation (Zolotarev, 1986), with the characteristic function and hence the density
and the distribution function jointly continuous in all four parameters, see the
right panel in Figure 1.3. In particular, percentiles and convergence to the
power-law tail vary in a continuous way as α and β vary. The location parame-
ters of the two representations are related by μ = μ0 — βσ tan ^πα for α = 1 and
μ = μ0 — βσ - ln σ for α = 1. Note also, that the traditional scale parameter
σG of the Gaussian distribution defined by:

^fG (^x ) = v2n^r_G ^exp ½^——     ¾^,               (1.4)

is not the same as σ in formulas (1.2) or (1.3). Namely, σG = -√^z2σ.

<<   3   4   5   6   7   8   9   >>

More intriguing information