Bird’s Eye View to Indonesian Mass Conflict Revisiting the Fact of Self-Organized Criticality

where

and theoretically we can say that the possible largest value of the casualties could always
increases in the limit of largest sample size.

3. Discussions: Self-Organization Criticality in Social Conflict and Massive Violence

Finally we arrive to the possible theoretical discussion based upon the question of
what we can learn from the findings of the power law in this specific Indonesian social
conflict. The notion of the self-organized criticality has been proposed in Cederman (2003)
that brought to us the consequence of the self-organized criticality system where the steady
linear input generates tensions inside the system that in turn lead to non-linear and delayed
output ranging from small events to huge ones (Bak, 1996). In return, theoretically speaking
the social conflict could also features the strong degree of path dependence - the very
sensitive macro system to the initial conditions of the social system, or roughly speaking the
historical pathways from micro to macro properties of the system.

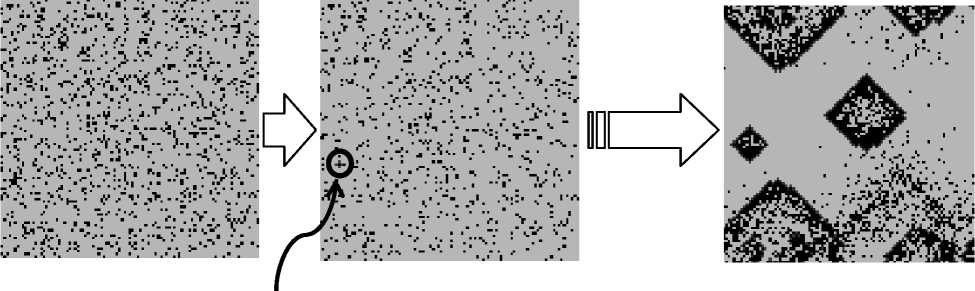
ignited fire

Figure 6. A running computational simulation of the classic forest fire model showing the self-organized
criticality could be an interesting analogical model to the conflict since
the size of the fire emerging the power law distribution
(cf. Drossel & Schwabl, 1992 and Turcotte, 1999)

That is probably why - as we have noted in the beginning of the paper - the massive
conflict and violence seemingly always brings surprise and sudden (felt) effect from the
peace to disrupting and harsh situations. Nonetheless, of course in the case of massive
conflict there are no regularities at all as in chaotic system (cf. Situngkir, 2002). A conjecture
to this theoretical understanding, however, has brought us to the discussions of the

<<   6   7   8   9   10   11   12   >>

More intriguing information