Chapter 4
Debye-HQckel equation (Eq. [4.17])[26]. Table 4.6 shows the effective diameter of
the ions in the synthetic brine [26].
Iog(Z) = -
0.509z,2√7
l + 0.328α,√/
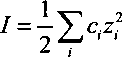
[4.17]
γi: activity coefficient of ion species /.
zi∖ valency of ion species /.
a,: effective diameter of the hydrated ion species /, in units of A.
I: ionic strength of the aqueous solution, in units of mol∕kg.
c∣: concentration of ion species /, in units of mol∕kg.
Table 4.7 shows the calculated activity coefficient of ions at the ionic strength
of synthetic brine.
4.3.3. Materials and methods
The aqueous phase used here is synthetic brine with pH 8.3, as introduced in
section 4.2.1. Kaolinite (A∣2Si2O5(OH)4) is obtained from Sigma-Aldrich with
detailed information in section 4.2.1. Alumina (AI2O3) is obtained from
Sigma-Aldrich (product #19944-3), with particle size 150 mesh (104 μm), pore
size 5.8 nm and specific surface area 155 m2∕g. All the salts in the synthetic brine
were obtained from Fisher Scientific.
All the samples of 50 ml 1.0 % (w∕w) kaolinite or alumina suspension were
119
More intriguing information
1. Ahorro y crecimiento: alguna evidencia para la economía argentina, 1970-20042. On s-additive robust representation of convex risk measures for unbounded financial positions in the presence of uncertainty about the market model
3. The name is absent
4. A Brief Introduction to the Guidance Theory of Representation
5. Palkkaneuvottelut ja työmarkkinat Pohjoismaissa ja Euroopassa
6. Partner Selection Criteria in Strategic Alliances When to Ally with Weak Partners
7. THE CHANGING STRUCTURE OF AGRICULTURE
8. The name is absent
9. XML PUBLISHING SOLUTIONS FOR A COMPANY
10. Volunteering and the Strategic Value of Ignorance