3370
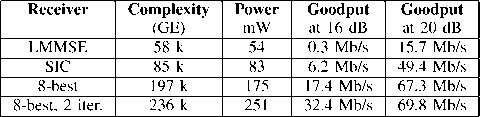
TABLE X
The Performance-Complexity Tradeoff With 2 × 2 16-QAM and
Detection Rate of 140 Mb/s
IEEE TRANSACTIONS ON SIGNAL PROCESSING, VOL. 58, NO. 6, JUNE 2010
4x4 antenna
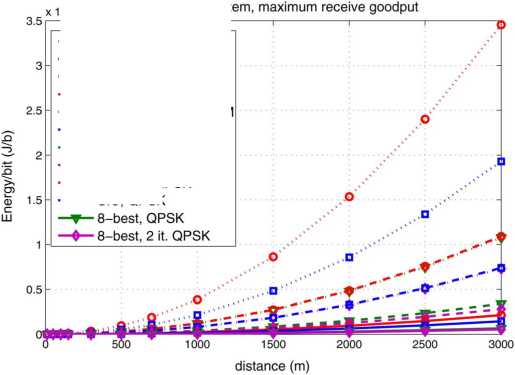
Fig. 16. The transmitter energy consumption per reliably transmitted bit versus
the propagation distance.
O LMMSE, 64-QAM
□ SIC, 64-QAM
V 16-best, 64-QAM
- θ- LMMSE, 16-QAM
0 16-best, 2 it, 64-QAM
- в - SIC, 16-QAM
- ▼ - 8-best, 16-QAM
- ♦ - 8-best, 2 it, 16-QAM
—θ- LMMSE, QPSK
—в—SIC, QPSK
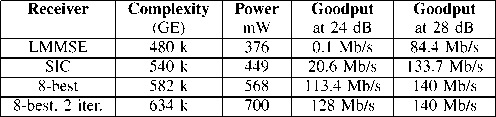
TABLE Xi
The Performance-Complexity Tradeoff With 4× 4 16-QAM and
Detection Rate of 280 Mb/s
(1 — FER)). The goodput now encapsulates performances of
both the implementation and the transmission.
The LMMSE receiver would require almost 10 dB more
transmit power in order to achieve the goodput of the A -bcst
LSD but the complexity is much higher with the K-best LSD.
The SIC receiver does not achieve the goodput of the RΓ-best
LSD but has a much lower complexity. In the 4 × 4 antenna
case, the SiC and LMMSE receivers do not give much goodput
at lower SNRs but all the receivers have a high goodput when
the SNR is high enough. if the channel is less correlated, the
receivers have a more similar performance and less transmit
power is needed to achieve a high goodput. The SiC receiver
produces the most bits per gate equivalent in the 2 × 2 antenna
system but at the 4 × 4 system, the R-best LSD is the most
efficient. A part of the performance degradation of the SiC
receiver in the 4 4 4 antenna system is due to the encoding of
the streams in LTE, which leads to interference cancellation
being performed between two layer pairs and the two layer
pairs being separated only by the LMMSE equalizer. The SiC
receiver would still provide a higher goodput than the LMMSE
receiver with a lower power consumption than the RΓ-best
LSD, thus offering a compromise between performance and
complexity.
As a final illustration of the performance-complexity trade-
off, the required transmit power to satisfy the rate and quality
requirements is considered in a simple example case. The power
efficiency of the transmitter in transmit power/transmission rate
in a 4 × 4 antenna system with a 20 MHz bandwidth is pre-
sented in Fig. 16. The needed transmit power was obtained from
Lw + AN + SNR + FM, where Lf is the free space path loss
20 log(4πd/λ), AN is the receiver noise floor (kTB + Receiver
noise figure of 6 dB) and FM is a 30-dB fade margin (Λ is the
wavelength, d is the distance from the base station, к is Boltz-
mann’s constant, T is the temperature [K] and B is the band-
width). The SNR is the signal strength at the receiver required
to achieve the maximum goodput. A simple path loss model
without shadowing or reflections was chosen for simplicity. The
required transmit power of the LMMSE receiver is at least twice
of that of the RΓ-best LSD. The transmit energy per bit grows
with the modulation order and is the same with 64-QAM RΓ-best
LSD and 16-QAM LMMSE. The ∕√-best LSD can receive data
reliably from a higher distance than the SiC or LMMSE receiver
with a fixed transmit power. With 4×4 64-QAM, the LMMSE
receiver can receive data at only very short distances with a rea-
sonable transmit power. Therefore, a more complex receiver is
needed to receive data from also larger distances.
Comparison of both performance and implementation to
literature can be difficult as the used channel models, methods
and the implementation technologies may be different. Many
of the implementations in the literature consider an uncoded
system or an uncorrelated channel, where also simple receivers
perform well, which can lead to different parameters in the
implementation. Depth-first sphere detector implementations
in the literature usually report an SNR dependent throughput
which makes the implementation comparison more chal-
lenging. The hard-output ∕2-norm JF-best detectors in [19] have
a lower complexity but were implemented with different list
sizes and CMoS technology from our work. The complexity
of the receiver increases by roughly 30% both in [19] and this
work when the list size is doubled. However, the detection rate
decreases almost five times in [19] but is only halved in our
work. The soft output JF-best implementation in [12] with a list
size of 5 in a 4 × 4 16-QAM system has almost a three times
lower throughput and half the complexity of our implementa-
tion with a list size 8. A soft output JF-best detector is presented
in [34]. It achieves a 50-Mb/s throughput with 4×4 64-QAM,
list size 256 and a silicon area of 20 mm2. The silicon area of
our implementation is four times smaller with the same CMoS
technology and the throughput is 210 Mb/s with a list size 16.
Vi. Conclusion
The performances and implementation complexities of the
LMMSE, SIC, noniterative and iterative RΓ-best LSD receivers
for MIMO-OFDM communications were compared. The
emphasis was on LTE specific system parameters and latency
requirements. The SIC receiver was shown to outperform the
TF-best LSD with horizontal encoding in channels with low
spatial correlation, but the result is reversed in channels with
significant spatial correlation; the SIC receiver is not practically
More intriguing information
1. The name is absent2. Perfect Regular Equilibrium
3. Does adult education at upper secondary level influence annual wage earnings?
4. Analyzing the Agricultural Trade Impacts of the Canada-Chile Free Trade Agreement
5. Implementation of the Ordinal Shapley Value for a three-agent economy
6. Cyclical Changes in Short-Run Earnings Mobility in Canada, 1982-1996
7. WP 1 - The first part-time economy in the world. Does it work?
8. Ein pragmatisierter Kalkul des naturlichen Schlieβens nebst Metatheorie
9. DIVERSITY OF RURAL PLACES - TEXAS
10. The name is absent