ci rel
20 г- Polynomial cointegration relation 1
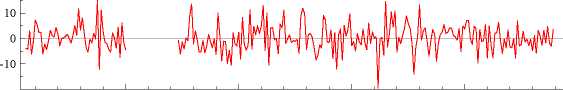
1980 1985 1990 1995
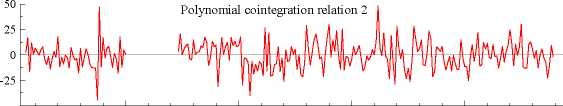
1980 1985 1990 1995
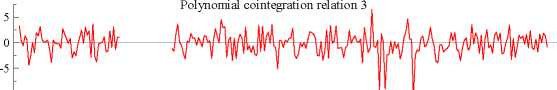
-10
3∙pdf 1980 1985 1990 1995
Figure 2: The graphs of the three polynomial cointegration relations. Upper panel
describes the IKE relation, the middel panel the inflation expectations relation, and
the lower panel the German inflation rate relation.
β'2xt + δ'2∆xt = {(½ - s2) + 0.60∆p2 - O.51∆pι - O.17∆sι2 + 0.00sι2 + 0.00⅛.1} (23)
The third relation, essentially a relation for German inflation rate, is similar to the
relation found in Juselius and MacDonald (2006) and describes the latter as (almost)
homogeneously related to US inflation rate, German short-term interest rate, and the
change in the Dmk/$ rate:
β3xt + J3∆xt = {1.31∆p1 - 0.31∆p2 - 0.74s1 - 0.07∆s12 + 0.00pp - 0.00t91.1} (24)
All three relations contain a tiny, but significant, trend effect which is more difficult
to interpret. The most likely explanation is the usual one that the linear trend effect in
the relations is a proxy for some information not included in the analysis. For example,
the small trend effect in (22) might account for some perceived productivity differential
between the two economies. In (23) the re-unification trend might be a proxy for a
change in the market’s re-assessment of the riskiness of the nominal Dmk/$ rate. In
(24) the trend together with the pp may imply that German inflation rate, in addition
to following the US inflation rate, the short-term interest rate, and the change in the
Dmk/$ rate, has exhibited a long-run adjustment to trend-adjusted relative prices.
Figure 2 shows that the three polynomially cointegrating relations are very stationary.
!
The β±11xt relations are CI(2,1) cointegrating relations which only become sta-
'
tionary by differencing. Thus, βx1∆xt could be interpretable as partially specified
20
More intriguing information
1. Meat Slaughter and Processing Plants’ Traceability Levels Evidence From Iowa2. The name is absent
3. The name is absent
4. Informal Labour and Credit Markets: A Survey.
5. Constructing the Phylomemetic Tree Case of Study: Indonesian Tradition-Inspired Buildings
6. Strategic Effects and Incentives in Multi-issue Bargaining Games
7. The name is absent
8. Opciones de política económica en el Perú 2011-2015
9. Peer Reviewed, Open Access, Free
10. The name is absent