Heterogeneity of Investors and Asset Pricing in a Risk-Value World

-fl (⅛)

-1

⁷^{- 2}^-^f '(⅛).

⁷^{- 1}^f^(eA ’

V i,ε

(33)

Hence

*>=7—1 ∑ (a+
i ×

⅛i

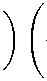
de_i ∕dε

A_i ■ —

^c I—7

)∙

(34)

Divide (30) by f (e_iε) and aggregate. This yields

¹ = -^τ'⁽^e⁾ ∑^-dif^fl⁽<Ы = ~'⁽^⁾ ∑ (a ⁺1—-) ⁽dJ^fiAA ⁽³⁵⁾

i i 7 ' /

Now divide (30) by f_i (⅛)∙ This and (35) yield

^fl ⁽<M
^fi ⁽A⁾

de_idε

^-'l. j(ɛ) =________^-dj^fi^(eA________

^fi ' ∑j ⅛√Λ∙(M (λ ⁺ ⅛)

(36)

The left hand side of this equation, multiplied by -1, equals the term in
the squared bracket of (34). Hence it follows that

7 ^{- 2} ^2^A⁺ 1-7________

⁷^{- 1 i} [∑J (½√^f,∙ ^(eie⁾)(^a⁺ f⅛)]

(⅛ )'

∀ ε.

(37)

Mne g_il ≡ (Г ⁺ ɪ^{/ /} l'^a⁺ ɪ)

V i, ε so that _i g-iε = 1 ^ ε.

Then (37) yields