Testing for One-Factor Models versus Stochastic Volatility Models

1 I<’^n-1>^rj (∑. =:; 1 {⅛∕n-,Y,∕n∣<M" U^/n (σ²(ɪ.) - σ²(Xi/n)) d«

∑n-1

j = 1 ¹ {∣ Xj∙∕n~X≈∕n∣<ξn}

~^∕""~

Dn,r

Now, given Lemma 1, D_n,_r = o_a._s. (1), provided that n¹ /²⁺^εξ_n → 0, as n → ∞. It is immediate
to see that H_n,_r is of a smaller order of probability than G_n,_r.

Let < G_n >_r denote the discretized quadratic variation process of F_n,_r. By a similar argument
as in Bandi and Phillips (2003, pp.271-272),
we have that

plim n→∞

< ^Gn >r

- 2 ∞ σ⁴ ( a )

-∞

L X ( r, a )²Lχ (1, a)

Thus, by the same argument as in the proof of Theorem 3 in Bandi and Phillips (2003), the
statement in Step 1 follows.

Proof of Step 2: It follows from Theorem 1 in Barndorff-Nielsen and Shephard (2004a).

Proof of Step 3: The discretized covariation process < A_n,B_n >_r,

< ^An, ^Bn >

L(n-1’rj [(n-1’rj

i =1 j =1

'¹ { ∣ .χ_iin-X∕,∣ <<n} ^J/N /n ( ' - ^χ∕n) σ(^χ. )^dW ² '
∑ⁿ=¹¹ { ∣ ^χ₃∕n-^χ_i∕n ∣ <ξn}

L(n-1’rj L(n-1’rj

= 2 n ∑ ∑

i =1 j =1

¹ { ∣ χ_j∕n-χ_i∕n ∣ <ξn}^σ⁴⁽^X^j/n⁺⁰^a-^s-⁽¹⁾⁾V^n-¹ m, , ,

²=1=1 ¹ {∣ Xj∕n-Xi∕n ∣ <ξn}

(19)

= 2

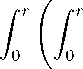
¹ {∖Xu-Xa∖<ξn}^σ⁴⁽^XU ^)d^UJθ¹¹ {∖Xu-Xa∖<ξn} ^d^U

d a + 0a.s. (1)

[∞ J ∣^,∞ 1 u a <ξ. σ⁴l∏lχ(∣: l∏<∖u

J-oo -J-OO J-∞ ¹ {∖u-a∖<ξn}LX⁽¹ ,^u^)d^u

Lx(r, a)da + o_a.s. (1),

where the 2 (instead of 4) on right hand side of (19) comes from Lemma 5.3 in Jacod and
Protter (1998). Along the lines of Bandi and Phillips (2001, 2003), by the change of variable

u-a

^ξn

= z,

< ^An, ^Bn >

CorPapers

Testing for One-Factor Models versus Stochastic Volatility Models

More intriguing information