Uncertain Productivity Growth and the Choice between FDI and Export

Uncertain Productivity Growth

However, the described relationship turns out to be idiosyncratic to linear profit functions. For
convex profit functions (κ > 1) an increase in productivity uncertainty does not only affect the
optimal cut-off level negatively (increase in tf*) but additionally exhibits a countervailing effect.
In such a case, the expected profits of both market entry modes rise, due to Jensen’s inequality
which reduces the optimal cut-off levels tf*. This positive adjustment is captured by the partial
differential of the adjusted discount rate

∂δu⁰              ₂

——■ = σκ — σκ² < 0.

∂σ

which is monotonically decreasing in σ . The intuition for this effect is that an investor can
expect higher profits associated with productivity changes and as a consequence the adjusted
discount rate increases the costs of waiting if σ increases. Therefore, for κ > 1 the total impact
of uncertainty on the expected market entry time depends on the modulus of the two effects. For

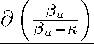
∂σ

the expected market entry time also increases in σ for κ > 1, however

due to the negative effect in δ_u⁰ .¹⁶

For specific parameter values, Jensen’s inequality dominates the total effect of an increase in
uncertainty and for such cases the expected market entry time can decrease. Plotting the expected
market entry time with respect to σ results therefore, in a u-shaped function (figure 9).

Differently expressed, for low levels of uncertainty the expected market entry time in both modes
decrease whereas for high levels of uncertainty, a shift in σ increases E(T_i*). Figure 9 shows that
for high productivity growth rates α the likeliness of a decrease in the expected market entry time
is higher than in cases with low growth rates. Technically, the range of values in which E(T_i*)
decreases in σ becomes bigger the higher the growth rate is (σ0 < σ1, in figure 9). The intuition
for this result is that companies associated with high growth rates may appreciate a certain
extent of productivity uncertainty and enter the market earlier. Whereas, firms confronted with

¹⁶ A detailed analysis can be found in Wong (2007).

35

<<   34   35   36   37   38   39   40   >>

More intriguing information