Stata Technical Bulletin
29
tiprel[Tenant]
• tiprel[Owner]
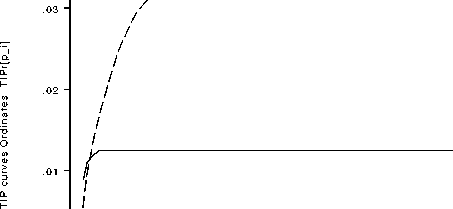
0 -
h-----------------------1-----------------------1-----------------------1-----------------------г
0 .25 .5 .75 1
Population Share, p_i
Three I's of Poverty Curves
Figure 5. TIP curves of relative poverty gaps.
References
Cowell, F. A. 1995. Measuring Inequality. 2d ed. Prentice-Hall/Harvester-Wheatsheaf, Hemel Hempstead.
Jenkins S. P. and P. J. Lambert. 1997. Three ‘I’s of poverty curves, with an analysis of UK poverty trends. Oxford Economic Papers 49: 317-327.
Lambert, P. J. 1993. The Distribution and Redistribution of Income—A Mathematical Analysis. 2d ed. Manchester University Press: Manchester and
New York.
Shorrocks, A. F. 1983. Ranking income distributions. Economica 197: 3-17.
sg108 Computing poverty indices
Philippe Van Kerm, GREBE, University of Namur, Belgium, [email protected]
Description
The objective of this insert is to help automate the estimation of a series of standard poverty measures from unit record
income data. The indices computed by poverty are classic measures from the Foster-Greer-Thorbecke class (including the
headcount ratio and the poverty gap ratio), the income gap ratio and the aggregate poverty gap, the Sen, Takayama, Thon
and Watts indices, and measures from the Clark-Hemming-Ulph class. The formulas for all these measures are given below.
However, I refer the reader to the literature on poverty measurement or to the original papers for an exposition of the properties
of the various indices (see among the references given below).
Consider a dataset of n observations with each entry being one income recipient unit (for example, household, individual,
and so on). Let yi be the income of the ith observation, u⅛ be the weight of the ith element (e.g., household size) r, be the
rank of the ith element in the whole distribution (taking weights into account), and z be the poverty line.
Define the indicator Ii = 0 if yi ≥ z, and 1 otherwise, and define N = ɪɪ ∣ u⅛, $ = ∑Γ=ι 'υ'∣^ι-
(Continued on next page)
More intriguing information
1. Mean Variance Optimization of Non-Linear Systems and Worst-case Analysis2. A Unified Model For Developmental Robotics
3. The name is absent
4. Monetary Discretion, Pricing Complementarity and Dynamic Multiple Equilibria
5. Two-Part Tax Controls for Forest Density and Rotation Time
6. The name is absent
7. Federal Tax-Transfer Policy and Intergovernmental Pre-Commitment
8. The name is absent
9. CREDIT SCORING, LOAN PRICING, AND FARM BUSINESS PERFORMANCE
10. Dual Track Reforms: With and Without Losers