Spectral calibration of exponential Lévy Models [1]

19

∆8

-U -U

+ E ^hl^{ll X} δkδiεkεiFbk (u)Fbi (v)l^{ll i´}

u ⁴ WU ( u ) v ⁴ WU ( v )

κ(u)²κ(v)^{2    du dv}

U U       ^N                       4U 4U

u W (u)v W (v)
∆    ∆⁸ + ∑ δkδj ∣Fb_k (u) |² ∣Fb_l (v) |² ----^{σ (} V) ₍ σ⁽ ) du

_{U -U   k i 1}                                 κ(u)²κ(v)²

k,i=1

^U
∆⁴

-U

u⁴W^U (u)    ^{2 U N} _{2        2} u⁴ W^U (u)    ²

₍\2 d u + / δLF^δbk F bk ( u ) 1²   (22 d u

κ(u)²              _{-U k=1}               κ(u)²

∆⁸U⁴+∆⁴U⁴∣δ∣i²2 E(Tσm²axU²)².

Putting all estimates together and using U .∆^-1 as well as∆∣δ∣_i²2 . ∣δ∣_i²∞
we obtain (32) and consequently the rate for σ² .

6.4. Upper bound for γ and λ

Since the claimed risk bound for γ is larger than for σ², we only need to
σ²

estimate the risk of γ + ^σ2- instead of that for γ. Equally, we can restrict
to λ - ^σ2— γ instead of λ. Then the proof follows exactly the lines of the
proof for σ², the only difference being the different norming in estimate
(30) giving rise to a factor U for γ and a factor U² for λ. It remains to
note that we obtain the bounds in the compound Poisson case by setting
σ = σmax = 0 and considering the continuous extension of the bounds for
that case. For γ we obtain as bias

lʃ F μ ( u ) WU ( u )d u∣ . U ^{- ( s}+²).

(41)

The linear error term is estimated by

U₂

E _-U L(u)Wγ^U(u) du .E(Tσ²U²) U²∆⁴+U∆∣δ∣i²∞

(42)

and the remainder satisfies

E

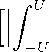
2

R(u)Wγ^U (u) du∣^∣ .E(Tσm²axU²)²∆⁸U⁶+∆⁴U⁶∣δ∣i²2 .

(43)

For λ we obtain the same asymptotic error bounds as for γ, but multiplied
by U when regarding the root mean square error. With the rate-optimal
choice (23) of U this gives the asserted risk bounds for ^ and λ.